 |  |
| (a) | (b) |



For a circular vortex defined by a tangential velocity uθ(r), the radial height/pressure profile is a solution of
| h′0(r)= |
| ⎛ ⎜ ⎜ ⎝ | 2Ω + |
| ⎞ ⎟ ⎟ ⎠ |
with Ω the angular velocity. For this test case we take
| uθ(r)=(r < 0.4)є(1+cos(π(r−0.2)/0.2))/2 |
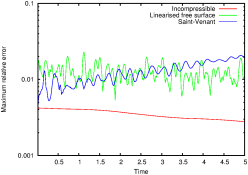
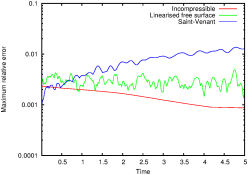
The control parameters are the Froude number U/√gH and the Rossby number Ro=U/Ω L. We set the Froude number to 0.1 and consider Rossby numbers 0.1 and ∞ (no rotation). In the case without rotation the errors reflect only the accuracy of the momentum advection terms. With rotation, the errors also depend on the accuracy of the discretisation of the geostrophic balance.
Figure 133 illustrate the evolution of the errors on free surface height/pressure for the different solvers, with and without rotation.
(a) (b)
Figure 134 display the error distributions at t=5.
incompressible linearised free-surface Saint-Venant


