This test case was proposed by LeVeque (JCP, 1998) as a check for the accuracy of hydrostatic balance for the Saint-Venant equations with variable topography. A solitary wave of small amplitude is generated by an initial discontinuity on the left-hand-side of the domain and moves past a parabolic hump creating complex focusing and diffraction (Figure 39). Any inaccuracy in hydrostatic balance will clearly affect the solution given the small amplitude of the initial perturbation.
Figure 39: Animation of the topography (coloured) and free surface (white). The vertical scale is exagerated. 
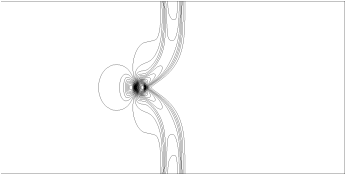
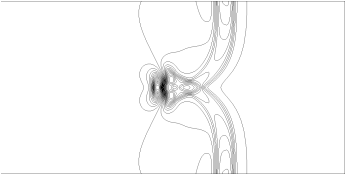
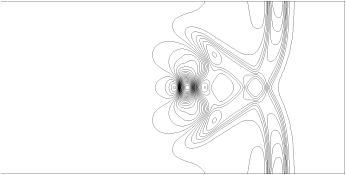
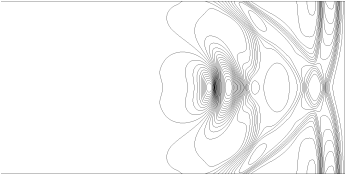
Figure 40 illustrates the free surface and corresponding adaptive mesh evolution. This figure agrees well with the results reported by LeVeque using a non-adaptive high-resolution Godunov method (Figure 7, right column, note that the resolution of the results by LeVeque is slightly larger: 600× 300 compared to 512× 256 here).
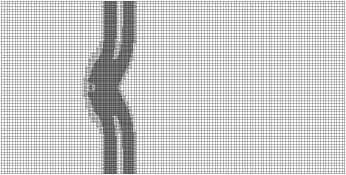t = 0.6 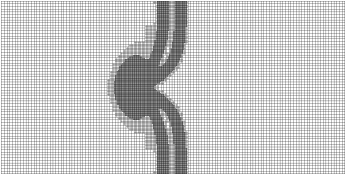t = 0.9 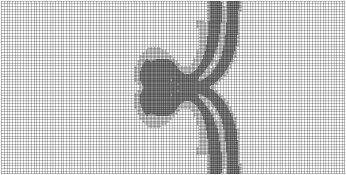t = 1.2 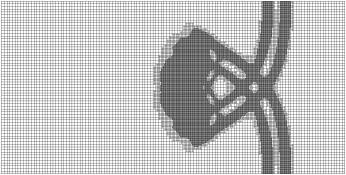t = 1.5 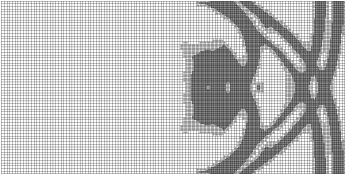t = 1.8
